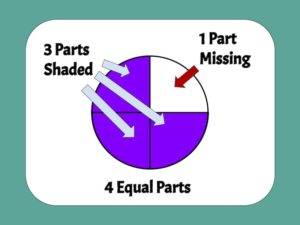
How to Teach Fractions: 4 Strategies for Student Success
Knowing how to teach fractions can make all the difference for student mastery. Follow these tips to build your students’ conceptual understanding.

My favorite math lesson ever is based on a little tool called The Sieve of Eratosthenes. (Pronounced: Siv of Air-a-tos-thin-ease).
It’s rare that a single math lesson can be used again and again, with students of different ages, while still having an impact. Either it’s too challenging for young students, or it’s boring for older students. And your students will definitely let you know when you teach them a lesson they learned the year before.
But here’s why I think the Sieve of Eratosthenes is different. In some ways, it’s just a glorified hundreds chart. But once you and your students start seeing the patterns in this hundreds chart, it gets really interesting. No matter how many patterns you find, there’s always another layer to be uncovered.
Finding patterns is all well and good, but what about concerns that students need to ‘focus on the basics?’ Here’s an interesting article from Forbes on the origins of the traditional vs conceptual debate.
Regardless of which camp you’re in, if you spend time in a math classroom, you know that students of any age can struggle with automaticity. I first realized the prevalence of this problem about 5 years ago, when I began working with schools on curriculum design.
Whenever I start working with a new school, we go over expected proficiencies for each grade level. I ask teachers to list what they expect from their incoming students, and what they expect from outgoing students.
Without fail, the 8th grade teachers feel that their students still don’t have their “math facts” down. They wonder why the 7th grade teachers never taught them. The 7th grade team points out that math facts aren’t even a 7th grade standard — it’s the 6th grade team’s fault, they contend. On and on this goes, each grade level wondering why the prior grade team hadn’t “covered” such an important topic.
The reality is that math facts get covered. And covered. Aaaand covered. Many high school teachers report that their students still need work on “the basics.”
Clearly the problem isn’t that we’re not teaching math facts. In fact, students are exposed to the content year after year, but for some reason it just isn’t sticking.
The term math facts is actually a pet peeve of mine. It makes me think of drills, repetitions, and timed quizzes. I prefer to think in terms of numeracy and fluency. If a student doesn’t know that 6×4 is 24, I want her to realize that it’s 4 more than 5×4. Even if it means skip counting or using her fingers, better to use conceptual strategies than sit down and memorize a list. A list she’ll forget as soon as the quiz is over.
Referring to fluency in terms of ‘facts’ just feels disconnected. I want students to become fluent by developing number sense. Addition is the opposite of subtraction. Multiplication is repeated addition. To count up by nines, increase the tens place by one, and reduce the ones place by one.
This is where the Sieve comes in. The act of coloring in patterns creates a powerful understanding of the connections. If I count up by 7 five times, I get to 35. Counting down from 35 by 7’s still takes 5 steps. Wow, I guess multiplication and division must be connected!
If I want to add 77+8, I can go 1 row down (+10) and two boxes left (-2). That’s a handy shortcut I’ll remember next time I’m doing mental math.
When students learn ‘facts’ in context, it makes the math more interesting. It’s also a powerful way to help them remember what they’ve learned — even after the next summer break.
 Outside of math class, a sieve is like a strainer – it’s used to separate liquids from solids. I use one regularly to remove water from spaghetti once it’s done cooking. In math, a sieve is a strategy or formula that “filters” numbers that don’t belong in a certain category.
Outside of math class, a sieve is like a strainer – it’s used to separate liquids from solids. I use one regularly to remove water from spaghetti once it’s done cooking. In math, a sieve is a strategy or formula that “filters” numbers that don’t belong in a certain category.
This particular sieve rules out composite numbers, leaving only primes. Eratosthenes’ innovation was to find prime numbers by process of elimination. Counting by 2’s up to 100, he could easily prove that 50 of those numbers were not prime. Next he would count up by 3’s, 5’s, 7’s, and so on. He skipped 4’s, 6’s and other composite numbers – any multiple of 4 or 6 had already been eliminated from consideration as they were multiples of 2.
Mathematicians spend a lot of time thinking about prime numbers. They play a role in factoring numbers and algebraic expressions, converting fractions, and so on. At the highest levels of mathematics, prime numbers are essential to cryptography. When you enter your credit card information on a website, prime numbers help ensure that no one but the intended recipient can see those digits.
As interesting as primes are, I’m a lot more interested in what the Sieve teaches students about multiplication and the relationships between numbers. This activity will have your students looking at multiplication in a whole new way. It’s an “open task,” meaning that each student will learn something unique.
For some, the sieve is a way to master times tables. For others, it will help with common denominators or factoring expressions. The sieve also highlights patterns that can improve mental math with all four operations.
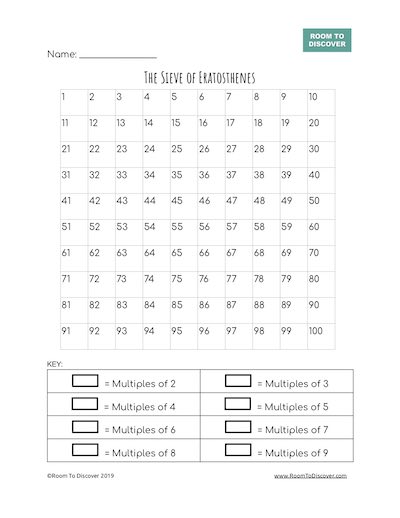
The Sieve of Eratosthenes is a fun and engaging lesson that will provide lasting benefits to students of any age. The basic idea is this: pick a color for each single digit number. (Eliminate 1 because it’s neither prime nor composite. Not to mention the ‘ones’ times tables aren’t very interesting or challenging). Draw a colored circle in the box for ‘2,’ and put a small ‘x’ of the same color in each multiple of two.
Once students finish drawing, you can build a rich discussion around the patterns they will find in the numbers. As you go through each multiple, different patterns arise and overlap with each other. Seeing the patterns visually will help to connect multiplication to division, and older students can use this as a tool to support factoring, LCM, GCF, and more.
If you’re ready to try this out with your class, you can download the worksheet and key from our online store.
You’ll also find the complete lesson, which includes historical background on Eratosthenes, a complete workshop-model lesson plan, notice & wonder graphic organizers, and a group work rubric.
Let us know how it goes – we’d love to see your students’ finished work in our Facebook group!

Knowing how to teach fractions can make all the difference for student mastery. Follow these tips to build your students’ conceptual understanding.

Many educators are starting to recognize the problem with homework. And while homework is almost universal, there is little evidence that it actually works.

Essential reading for every math teacher! The meanings of multiplication support student engagement, conceptual understanding, and success with word problems.